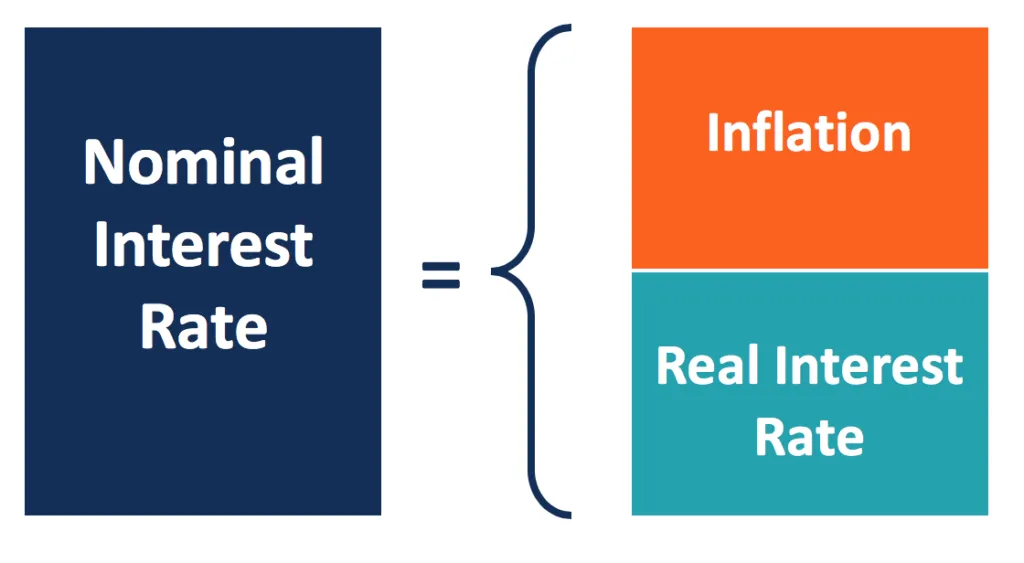
The distinction between nominal and real interest rates is a fundamental concept in economics, finance, and investing. Both rates are used to describe the interest on loans or the return on investments, but they differ in how they account for inflation.
### Nominal Interest Rate
The nominal interest rate is the stated rate on a loan or investment without adjusting for inflation. It represents the rate of interest charged by lenders or the rate of return promised by an investment before considering the eroding effects of inflation on purchasing power. Essentially, it’s the “face value” rate that does not take into account the real value of money over time. For example, if a bank offers a savings account with a 5% nominal interest rate, this rate does not reflect the changing value of money.
### Real Interest Rate
The real interest rate, on the other hand, is the nominal interest rate adjusted for inflation, reflecting the true purchasing power of the interest payments or investment returns. It gives a more accurate picture of the lender’s true earnings or the investor’s real rate of return. The real interest rate can be calculated using the Fisher Equation, which is:
\[
\text{Real Interest Rate} = \frac{1 + \text{Nominal Interest Rate}}{1 + \text{Inflation Rate}} – 1
\]
Alternatively, for small rates, a simpler approximation can be used:
\[
\text{Real Interest Rate} = \text{Nominal Interest Rate} – \text{Inflation Rate}
\]
The real interest rate is crucial for making informed decisions about lending, borrowing, and investing. If the real interest rate is positive, money or capital gains purchasing power over time. If it’s negative, the purchasing power decreases.
### Importance in the Economy
Understanding the difference between nominal and real interest rates is essential for several reasons:
– **Investment Decisions**: Investors look at the real interest rate to determine the real growth of their investments after adjusting for inflation.
– **Economic Policy**: Central banks monitor real interest rates closely when setting monetary policy to control inflation and influence economic growth.
– **Financial Planning**: For individuals, understanding the difference helps in making more informed decisions about loans and investments, ensuring their savings and investments grow in real terms.
In summary, while the nominal interest rate tells you how much your investment will grow in nominal terms, the real interest rate tells you how much your investment’s purchasing power will grow, which is critical for long-term financial planning and analysis.
Let’s see two examples:
**Problem 1:**
If the real interest rate is 2% and the inflation rate is 3%, what is the nominal interest rate?
**Solution:**
Using the formula:
\[ \text{Nominal Interest Rate} = \text{Real Interest Rate} + \text{Inflation Rate} \]
\[ \text{Nominal Interest Rate} = 2\% + 3\% = 5\% \]
So, the nominal interest rate is 5%.
**Problem 2:**
John is planning to buy a house and is considering a mortgage loan. The real interest rate on the mortgage is 2.5% annually. The inflation rate is expected to be 3% annually. Calculate the nominal interest rate for the mortgage. Additionally, if John plans to pay off his mortgage in 20 years, calculate the total interest paid over the loan period, considering the inflation rate remains constant.
**Solution:**
1. **Calculate the Nominal Interest Rate:**
Using the formula:
\[ \text{Nominal Interest Rate} = \text{Real Interest Rate} + \text{Inflation Rate} \]
\[ \text{Nominal Interest Rate} = 2.5\% + 3\% = 5.5\% \]
2. **Calculate the Total Interest Paid Over 20 Years:**
Assume John takes a loan of $200,000 with a nominal interest rate of 5.5%.
Using the mortgage formula:
\[ M = P \frac{r(1+r)^n}{(1+r)^n – 1} \]
Where:
– \( M \) is the monthly payment
– \( P \) is the loan amount ($200,000)
– \( r \) is the monthly interest rate (5.5% / 12 months = 0.004583)
– \( n \) is the number of payments (20 years * 12 months = 240)
\[ M = 200,000 \frac{0.004583(1+0.004583)^{240}}{(1+0.004583)^{240} – 1} \]
\[ M \approx 1,376.30 \]
Total payments over 20 years:
\[ 1,376.30 \times 240 = 330,312 \]
Total interest paid:
\[ 330,312 – 200,000 = 130,312 \]
So, John will pay approximately $130,312 in interest over 20 years.