Monopoly Cost, Revenue, and Profit – Exercise

Monopoly Cost, Revenue, and Profit

A monopolistic firm faces the following marginal revenue function:

$$MR(Q) = -10 + 7Q$$

and the marginal cost function:

$$MC(Q) = 20 – 14Q + 3Q^2$$

The firm has a fixed operating cost of 100 currency units and receives a government subsidy of 140 currency units (independent of production). The firm has no other revenue or costs beyond the sale of its product.

Questions

(a) Determine the total cost function $TC(Q)$ and the average cost function $AC(Q)$.
(b) Determine the total revenue function $TR(Q)$.
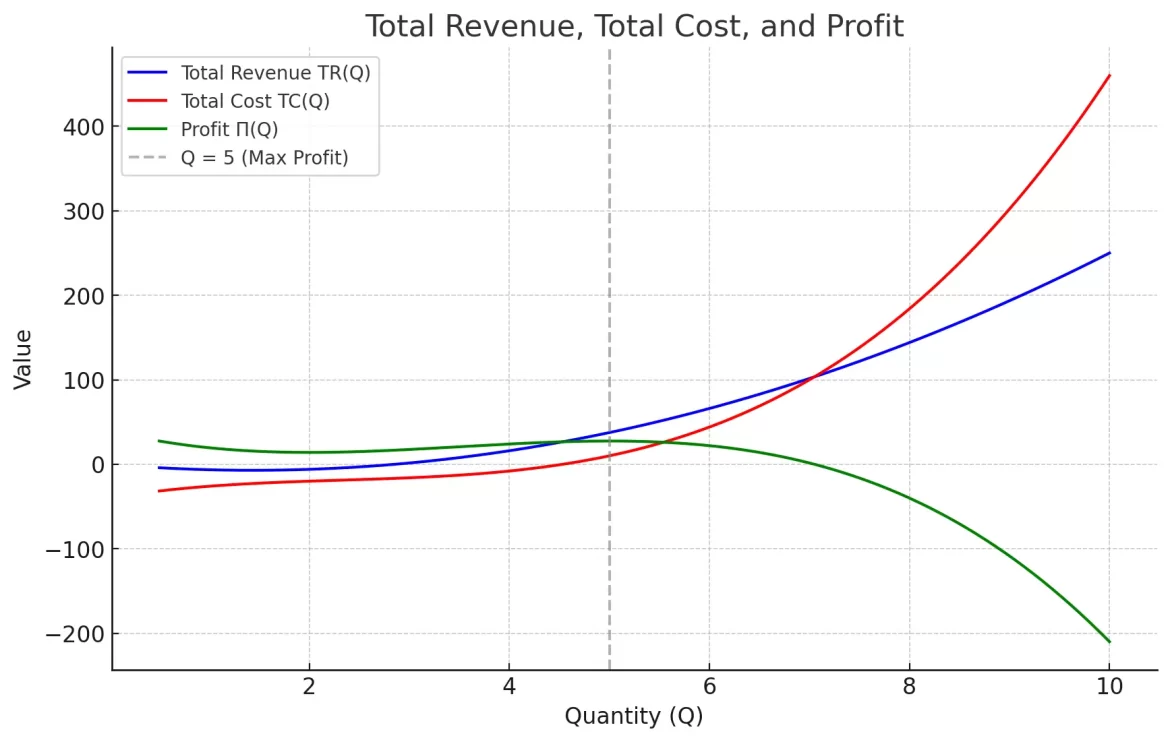
(c) Determine the total profit function $\Pi(Q)$.
(d) Find the quantity of output $Q$ that maximizes the firm’s profit.

Solutions

(a) Total Cost and Average Cost

We are given the marginal cost function:

$$MC(Q) = 20 – 14Q + 3Q^2$$

To find the total cost function, integrate $MC(Q)$ with respect to $Q$:

$$TC(Q) = \int MC(Q) \, dQ = \int (20 – 14Q + 3Q^2) \, dQ$$ $$TC(Q) = 20Q – 7Q^2 + Q^3 + C$$

The fixed cost is 100 and the firm receives a subsidy of 140. That gives a net fixed cost of:

$$C = 100 – 140 = -40$$

So the total cost function is:

$$TC(Q) = Q^3 – 7Q^2 + 20Q – 40$$

The average cost function is:

$$AC(Q) = \frac{TC(Q)}{Q} = Q^2 – 7Q + 20 – \frac{40}{Q}$$

(b) Total Revenue

We are given the marginal revenue function:

$$MR(Q) = -10 + 7Q$$

Integrate to get total revenue:

$$TR(Q) = \int MR(Q) \, dQ = \int (-10 + 7Q) \, dQ = -10Q + \frac{7}{2}Q^2 + C$$

Since revenue is zero when $Q = 0$, $C = 0$. So:

$$TR(Q) = -10Q + \frac{7}{2}Q^2$$

(c) Total Profit Function

Profit is revenue minus cost:

$$\Pi(Q) = TR(Q) – TC(Q)$$ $$\Pi(Q) = \left(-10Q + \frac{7}{2}Q^2\right) – \left(Q^3 – 7Q^2 + 20Q – 40\right)$$

Simplify:

$$\Pi(Q) = -10Q + \frac{7}{2}Q^2 – Q^3 + 7Q^2 – 20Q + 40$$ $$\Pi(Q) = -Q^3 + \left(\frac{7}{2} + 7\right)Q^2 – 30Q + 40$$ $$\Pi(Q) = -Q^3 + \frac{21}{2}Q^2 – 30Q + 40$$

(d) Profit Maximization

To find the quantity that maximizes profit, take the derivative of the profit function and set it to 0:

$$\frac{d\Pi}{dQ} = -3Q^2 + \frac{42}{2}Q – 30 = -3Q^2 + 21Q – 30$$

Solve:

$$-3Q^2 + 21Q – 30 = 0$$

Divide through by -3:

$$Q^2 – 7Q + 10 = 0$$ $$Q = \frac{7 \pm \sqrt{(-7)^2 – 4(1)(10)}}{2(1)} = \frac{7 \pm \sqrt{49 – 40}}{2} = \frac{7 \pm 3}{2}$$ $$Q = 5 \quad \text{or} \quad Q = 2$$

To determine which gives a maximum, evaluate the second derivative:

$$\frac{d^2\Pi}{dQ^2} = -6Q + 21$$

• At $Q = 2$: $-6(2) + 21 = 9 > 0$ → local minimum
• At $Q = 5$: $-6(5) + 21 = -9 < 0$ → local maximum

✅ The firm maximizes profit at $Q = 5$ units of output.

Summary

• Total Cost: $TC(Q) = Q^3 – 7Q^2 + 20Q – 40$
• Average Cost: $AC(Q) = Q^2 – 7Q + 20 – \frac{40}{Q}$
• Total Revenue: $TR(Q) = -10Q + \frac{7}{2}Q^2$
• Profit: $\Pi(Q) = -Q^3 + \frac{21}{2}Q^2 – 30Q + 40$
• Maximum profit is achieved when $Q = 5$